Ambiguous Case Law Of Cosines
| We have seen combinations of sides and angles associated with the criteria for congruent triangles.
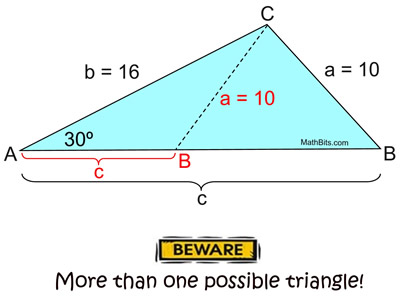
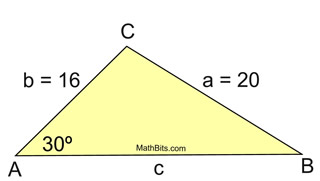
These combinations are at present going to show us when to use the Law of Sines and Law of Cosines. Using Law of Cosines with SSA We accept seen that using the Police of Cosines with the combinations SAS and SSS guarantees 1 unique solution and 1 unique triangle. Working with the third option of SSA, withal, continues to leave the door open for several different situations to occur, as information technology did with the Police of Sines. SSA is withal referred to as the Ambiguous Instance. Unlike the Ambiguous Case for the Law of Sines, the Ambiguous Case associated with the Law of Cosines will always require the solution to a quadratic equation to find a missing side. SSA : If two sides and the non-included angle are given, three situations may occur.
To summarize the Ambiguous Case: The solution(s) to the quadratic equation tell you the needed information: Notation: The re-posting of materials (in part or whole) from this site to the Net is copyright violation | |||||||||||||||||||||||||
Ambiguous Case Law Of Cosines,
Source: https://mathbitsnotebook.com/Geometry/TrigApps/TAUsingLawCosines.html
Posted by: petersonhadioncoulne1959.blogspot.com


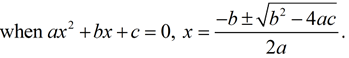



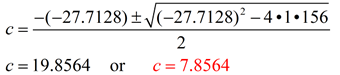


0 Response to "Ambiguous Case Law Of Cosines"
Post a Comment